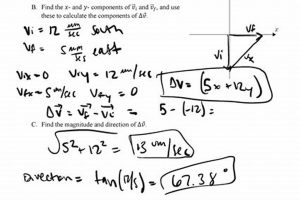
The Greek letter (uppercase) or (lowercase) frequently appears in physics examinations and related crossword puzzles. It typically represents angular velocity, a crucial concept in rotational motion. For example, a question might ask for the angular velocity of a spinning wheel given its rotational frequency.
Understanding angular velocity is fundamental to comprehending numerous physics concepts, including torque, rotational kinetic energy, and angular momentum. Its presence on tests underscores its significance within the field. Historically, the symbol’s adoption stems from its connection to circular motion and its mathematical relationship to frequency and period. This representation has become standard, simplifying communication and problem-solving in physics.
Further exploration of related concepts, such as rotational kinematics and dynamics, will provide a more comprehensive understanding of rotational motion and its applications.
Tips for Handling Angular Velocity Problems
Successfully navigating physics problems involving angular velocity requires a clear understanding of the underlying concepts and effective problem-solving strategies. The following tips offer guidance for approaching these challenges.
Tip 1: Understand the Relationship Between Angular Velocity and Frequency: Angular velocity () and frequency (f) are closely related. = 2f. Recognizing this connection simplifies conversions between these quantities.
Tip 2: Pay Attention to Units: Angular velocity is typically measured in radians per second (rad/s). Ensuring consistent units throughout calculations is crucial for accurate results.
Tip 3: Visualize the Rotation: Creating a mental picture of the rotating object can aid in understanding the direction and magnitude of the angular velocity.
Tip 4: Differentiate Between Angular Velocity and Linear Velocity: While related, these are distinct concepts. Linear velocity describes the speed of a point on the rotating object, while angular velocity describes the rate of rotation.
Tip 5: Utilize the Right-Hand Rule: The right-hand rule helps determine the direction of the angular velocity vector based on the direction of rotation.
Tip 6: Practice with Varied Problems: Exposure to different problem types reinforces understanding and improves problem-solving skills. Working through examples involving different scenarios, such as constant angular velocity and angular acceleration, builds proficiency.
Tip 7: Review Relevant Equations: Familiarize yourself with equations related to angular velocity, such as those for rotational kinetic energy and angular momentum.
Mastering these concepts and techniques will significantly enhance one’s ability to analyze and solve problems involving angular velocity, leading to a stronger grasp of rotational motion principles.
By applying these tips, individuals can develop a comprehensive understanding of angular velocity and its role in physics.
1. Angular Velocity
Angular velocity holds a prominent position in physics, particularly within the realm of rotational motion. Its representation by the Greek letter omega () makes it a frequent topic in physics examinations, often appearing in crossword puzzles as a conceptual clue. Understanding the facets of angular velocity is essential for solving related problems and deciphering its meaning in such contexts.
- Definition and Units
Angular velocity quantifies the rate of change of angular displacement. It represents how quickly an object rotates around a specific axis. The standard unit of measurement is radians per second (rad/s). In a crossword puzzle, the clue might refer to “radians per second” or “rotational speed,” pointing towards “omega” as the solution.
- Relationship to Frequency
Angular velocity is directly proportional to frequency (f), the number of rotations per unit of time. The equation = 2f demonstrates this connection. A crossword could exploit this relationship, using a clue like “Two pi times frequency” to lead solvers to “omega.”
- Vector Nature
Angular velocity possesses both magnitude and direction, classifying it as a vector quantity. The direction is determined by the right-hand rule, where the curled fingers point in the direction of rotation, and the thumb indicates the vector’s direction. While less common in basic crosswords, advanced physics puzzles might incorporate vector properties into clues.
- Applications in Physics Problems
Angular velocity plays a critical role in various physics calculations. It’s crucial for determining rotational kinetic energy, angular momentum, and torque. A crossword puzzle might indirectly reference these concepts, providing a context clue related to rotational motion to guide solvers towards “omega.”
These aspects collectively illustrate the importance of angular velocity in physics. Its representation as “omega” on a physics test crossword underscores its significance as a fundamental concept in rotational motion. Understanding its definition, units, relationship to frequency, vector nature, and applications equips individuals to tackle related problems and successfully navigate physics assessments, including deciphering crossword clues.
2. Rotational Motion
Rotational motion, the movement of an object around an axis, is intrinsically linked to the concept of angular velocity, often represented by the Greek letter omega () in physics. This connection makes “omega” a frequent entry in physics-related crossword puzzles, particularly those focused on motion. Understanding rotational motion is crucial for grasping the significance of omega in such contexts. The relationship stems from the definition of angular velocity itselfthe rate of change of angular displacement. Essentially, omega quantifies how quickly an object spins. This direct relationship underscores the importance of rotational motion as a foundational component of understanding angular velocity.
Real-world examples solidify this connection. Consider a spinning bicycle wheel. Its rotational motion is characterized by its angular velocity. A higher omega indicates a faster spin. Similarly, the Earth’s rotation about its axis possesses an angular velocity. These examples illustrate the practical significance of comprehending the link between rotational motion and angular velocity. This understanding allows for the analysis and prediction of rotational behavior in various systems, from simple machines to celestial bodies. The ability to calculate omega provides insights into the dynamics of rotating systems.
In summary, rotational motion forms the basis for understanding and applying the concept of angular velocity. The symbol omega, frequently encountered in physics test crosswords, serves as a constant reminder of this fundamental relationship. Challenges in comprehending rotational motion often translate into difficulties interpreting and applying omega in problem-solving scenarios. Mastering this connection is essential for a thorough understanding of rotational dynamics and their applications in various fields of physics and engineering.
3. Radians per second
Radians per second (rad/s) serves as the standard unit for angular velocity, symbolized by omega (), a concept frequently encountered in physics assessments, including crosswords. This unit signifies the rate of change of angular displacement, expressing how quickly an object rotates. The connection is fundamental: omega represents the magnitude of angular velocity, while radians per second provides the quantifiable measure. Understanding this relationship is crucial for correctly interpreting and solving physics problems involving rotational motion. For instance, an omega of 2 rad/s indicates one full rotation per second. Without specifying “radians per second,” the numerical value of omega lacks physical meaning in the context of rotational motion.
The importance of radians per second extends beyond simply providing units. It clarifies the nature of the rotation. Consider two objects with the same numerical omega but different units: one in rad/s and the other in degrees per second. The former uses radians, a dimensionless unit related to the circumference of a circle, providing a direct link to the angular displacement. The latter relies on degrees, an arbitrary division of a circle. This distinction impacts calculations involving angular displacement, arc length, and other related quantities. For example, calculating the linear speed of a point on a rotating object requires omega in rad/s to ensure consistency with the definition of radians.
In summary, “radians per second” is not merely an appendage to omega; it defines the physical meaning and allows for proper calculations involving rotational motion. Challenges in understanding this connection can lead to misinterpretations of omega and subsequent errors in problem-solving. A solid grasp of this relationship enhances one’s ability to analyze rotational motion and contributes to success in physics assessments, including deciphering crossword clues related to angular velocity.
4. Frequency's Counterpart
Within the context of a physics test crossword, “frequency’s counterpart” often alludes to angular velocity (), commonly represented by the Greek letter omega. This connection stems from the close mathematical relationship between the two concepts, making omega a likely solution when frequency is mentioned in a crossword clue. Understanding this relationship is crucial for solving such puzzles and for grasping the broader concepts of rotational motion.
- The Mathematical Link
The relationship between angular velocity () and frequency (f) is expressed by the equation = 2f. Frequency denotes the number of complete oscillations or rotations per unit of time, typically measured in Hertz (Hz). Angular velocity, on the other hand, quantifies the rate of change of angular displacement, measured in radians per second (rad/s). The equation highlights that angular velocity is directly proportional to frequency, with the constant of proportionality being 2. This direct link makes them counterparts in describing rotational motion.
- Implications for Crossword Puzzles
This mathematical relationship frequently appears in physics-themed crosswords. Clues might refer to “frequency’s rotational counterpart,” “2 times frequency,” or similar phrases, all pointing towards omega as the solution. Recognizing this link is essential for successfully completing such puzzles.
- Real-World Examples
Consider a spinning wheel. Its frequency describes how many times it completes a full rotation per second. Its angular velocity, however, describes how quickly its angle changes, providing a more nuanced understanding of its rotational motion. This distinction is relevant in various applications, including machinery design and analysis of rotating systems.
- Conceptual Understanding
While mathematically linked, frequency and angular velocity offer different perspectives on rotational motion. Frequency focuses on the number of cycles, while angular velocity focuses on the rate of change of angular position. Understanding this distinction enhances comprehension of rotational dynamics and facilitates problem-solving in physics.
In conclusion, understanding the relationship between frequency and angular velocity, its “counterpart,” is fundamental for navigating physics-related challenges, including crossword puzzles. The connection highlighted by the equation = 2f underscores their intertwined roles in describing rotational motion. This knowledge equips individuals to interpret clues effectively and solve problems related to this key concept in physics.
5. Scalar or Vector
The nature of omega (), representing angular velocity, as either a scalar or vector quantity directly impacts its interpretation within a physics test crossword and, more broadly, in physics problem-solving. While often treated as a scalar in simpler scenarios, especially in introductory physics and basic crosswords, omega possesses both magnitude and direction, inherently making it a vector. This distinction becomes crucial when analyzing more complex rotational motion, particularly involving three-dimensional rotation and the application of the right-hand rule. A crossword puzzle might use clues like “direction of rotation” or “axial vector” to indicate omega’s vector nature, increasing the complexity and requiring a deeper understanding of the concept.
Consider a spinning top. Its angular velocity can be described by a scalar value (e.g., 10 rad/s) if only the magnitude of the spin is considered. However, to fully describe the top’s motion, the direction of the rotation axis must also be specified. This requires treating omega as a vector, where its direction is determined by the right-hand rule: fingers curl in the direction of rotation, and the thumb points along the axis, indicating the vector direction. The vector nature of omega becomes critical when analyzing precession or when dealing with multiple rotating components within a system. For instance, the angular momentum of a rotating object depends on the vector nature of omega.
In summary, whether omega is treated as a scalar or a vector depends on the complexity of the rotational motion being analyzed. While introductory physics and simple crossword puzzles might focus on the scalar aspect, a thorough understanding of rotational dynamics requires recognizing omega’s inherent vector properties. This distinction significantly impacts problem-solving approaches in physics and influences the interpretation of related crossword clues, demonstrating the importance of considering both magnitude and direction when analyzing rotational motion.
6. Crossword Clue
The presence of “omega” on a physics test crossword hinges on its function as a crossword clue. Crossword clues provide hints or definitions that guide solvers toward specific answers. In the context of physics, “omega” acts as a critical link between the symbolic representation of a physical quantity (angular velocity) and its conceptual understanding. The clue itself might take various forms, ranging from direct definitions (“Angular velocity symbol”) to more intricate descriptions involving related concepts like frequency or rotational motion (“Frequency’s counterpart,” “Rotational speed”). The effectiveness of a crossword clue depends on its ability to evoke the relevant physics knowledge while adhering to the constraints of the puzzle format.
Consider a crossword puzzle featuring the clue “Unit of angular velocity.” This clue directly targets the concept of angular velocity and requires the solver to recall its unit of measurement, leading them to “rad/s” (radians per second) as the answer. A more challenging clue might be “2f,” requiring the solver to recognize the mathematical relationship between frequency (f) and angular velocity (), where = 2f. Such clues test not only recall but also the application of formulas and concepts. Real-world examples rarely involve explicit crossword puzzles but highlight the broader importance of clear and concise definitions in scientific communication. Just as a well-crafted crossword clue guides the solver to the correct answer, precise terminology and definitions in physics facilitate accurate communication and problem-solving.
In summary, the role of “crossword clue” in the context of “omega on a physics test crossword” underscores the importance of concise and effective communication in physics education and assessment. Well-constructed clues bridge the gap between symbolic representations, formulas, and conceptual understanding. Challenges in interpreting these clues often reflect gaps in understanding the underlying physics concepts. Therefore, analyzing “omega” as a crossword clue provides valuable insights into the pedagogical aspects of physics education, emphasizing the importance of clear definitions and effective communication strategies.
7. Physics Concept
The intersection of “physics concept” and “omega on a physics test crossword” highlights the pedagogical use of crosswords in reinforcing fundamental physics principles. “Omega,” representing angular velocity, serves as a focal point, connecting the symbolic representation to the underlying physical phenomenon. This approach reinforces learning by requiring students to recall and apply their understanding of angular velocity within the constraints of the crossword format. The effectiveness of this method relies on the crossword clue’s ability to evoke the relevant physics concept, prompting students to retrieve and utilize their knowledge. A well-crafted clue acts as a trigger, stimulating recall and application of the concept, strengthening the link between the symbol and its meaning.
Consider the physics concept of rotational kinetic energy. A crossword puzzle might feature a clue like “Energy of a spinning object,” requiring students to connect this concept to its formula, which involves omega (angular velocity). This reinforces the importance of omega in understanding and calculating rotational kinetic energy. Another example involves the relationship between angular velocity and linear velocity. A clue like “v = r” prompts students to recall this crucial equation, further solidifying their understanding of omega’s role in connecting rotational and linear motion. Real-world applications, such as designing rotating machinery or analyzing planetary motion, rely on a solid grasp of these concepts, demonstrating the practical significance of this understanding.
In summary, the inclusion of “omega” in a physics test crossword underscores the importance of conceptual understanding in physics education. The crossword format provides an engaging and effective method for reinforcing key concepts like angular velocity, connecting symbolic representations to real-world applications. Challenges in interpreting omega-related clues often indicate gaps in conceptual understanding, highlighting areas where further instruction or practice is needed. This approach strengthens the link between theory and application, contributing to a deeper understanding of physics principles.
Frequently Asked Questions about Omega in Physics
This section addresses common queries regarding the symbol omega () and its significance in physics, particularly within the context of rotational motion.
Question 1: What physical quantity does omega represent in physics?
Omega typically represents angular velocity, a measure of how quickly an object rotates around an axis.
Question 2: How is angular velocity different from linear velocity?
Linear velocity describes the rate of change of an object’s position along a straight path, while angular velocity describes its rotation around an axis.
Question 3: What are the standard units for angular velocity?
Angular velocity is typically measured in radians per second (rad/s).
Question 4: How is angular velocity related to frequency?
Angular velocity () and frequency (f) are related by the equation = 2f, where f is measured in Hertz (cycles per second).
Question 5: Is omega a scalar or a vector quantity?
Omega is inherently a vector quantity as it possesses both magnitude and direction. The direction is determined by the right-hand rule, aligned with the axis of rotation.
Question 6: Why is omega important in physics?
Angular velocity is fundamental to understanding and calculating various aspects of rotational motion, including rotational kinetic energy, angular momentum, and torque. Its significance extends to fields like mechanics, electromagnetism, and astronomy.
Understanding these frequently asked questions about omega enhances one’s comprehension of rotational motion and its importance in diverse areas of physics.
Further exploration of related topics, including rotational dynamics and the applications of angular velocity, will provide a more comprehensive understanding of this crucial concept.
Conclusion
This exploration has delved into the significance of “omega” within the context of a physics test crossword, highlighting its multifaceted nature. The analysis encompassed its representation of angular velocity, a crucial concept in rotational motion, its relationship to frequency, its units of radians per second, and its inherent vector properties. The discussion emphasized the importance of understanding these aspects not only for solving crossword puzzles but also for comprehending broader physics principles. The examination of “omega” as both a scalar and vector quantity underscored the need for a nuanced understanding of its role in different problem-solving scenarios.
A strong grasp of angular velocity, symbolized by omega, is fundamental for anyone pursuing a deeper understanding of physics. Further exploration of related concepts, such as rotational dynamics, torque, and angular momentum, will provide a more comprehensive appreciation of the intricacies of rotational motion and its widespread applications in various scientific and engineering disciplines. Continued study and problem-solving practice will solidify this understanding, fostering a greater appreciation for the elegant and powerful principles governing the physical world.